Exploring Degrees of Freedom: From Mechanics to Robotics

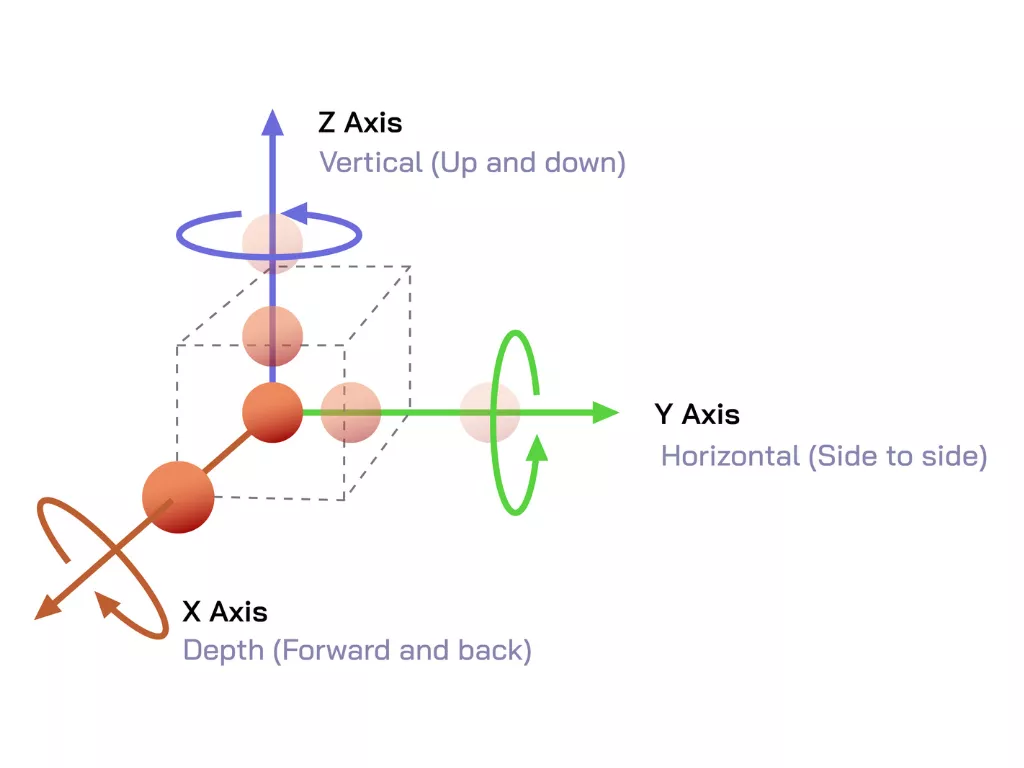
The concept of Degree of Freedom (DOF) is fundamental in fields such as physics, engineering, and robotics. It refers to the number of independent parameters that define the configuration or state of a mechanical system. Essentially, DOF indicates how many ways a system can move or be manipulated. 

To demonstrate the idea of DOF, we can calculate the degrees of freedom of a human hand. The human hand is a wonder of biological engineering; it is a complicated device capable of performing a wide range of movements and jobs with great dexterity and precision. This versatility is primarily due to its unique structure and the intricate interplay of its various parts. The degree of freedom of the hand is an important aspect in determining its capabilities.

In this blog, we will look at what degrees of freedom are, how they apply to the human hand, and why the hand's degrees of freedom are such a feat of engineering.